Maths vocabulary
- Mr Bee

- Dec 2, 2023
- 2 min read
Mathematics is a language and culture in its own right, and just like any language, it has its own specific and technical vocabulary. Understanding mathematical terms and their relationships is crucial in mastering the fundamental operations: addition, subtraction, multiplication, and division. Teaching these concepts to children not only involves teaching the operations themselves but also the associated vocabulary. Let's delve into how understanding mathematical terms aids in reasoning within each operation.
Addition: Exploring Addends and Sums
Addends + Addend = Sum
Addition is about combining quantities. The numbers we add are called addends, and the result is known as the sum. For instance, 2 + 3 = 5, where 2 and 3 are addends, and 5 is the sum.
How Vocabulary Aids Reasoning: When children understand that changing an addend affects the sum, they grasp the concept better. Adding 1 to an addend means adding 1 to the sum, reinforcing the idea that every change in an addend influences the final outcome.

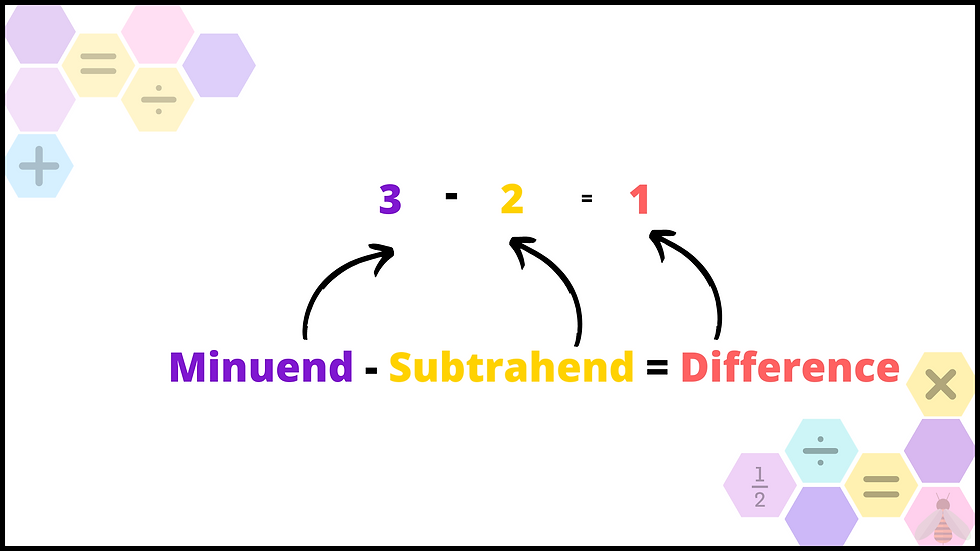
Subtraction: Understanding Minuends, Subtrahends, and Differences
Minuend - Subtrahend = Difference
Subtraction involves taking away one quantity from another. The starting number is the minuend, the number being subtracted is the subtrahend, and the result is the difference. For instance, 7 - 4 = 3, where 7 is the minuend, 4 is the subtrahend, and 3 is the difference.
How Vocabulary Aids Reasoning: Knowing the terminology helps children understand that when they subtract, they are finding the difference between two numbers. This understanding empowers them to tackle word problems and real-life scenarios.
Multiplication: Embracing Factors and Products
Factor x Factor = Product
Multiplication involves repeated addition or scaling of numbers. The numbers being multiplied are factors, and the result is the product. For example, 2 × 4 = 8, where 2 and 4 are factors, and 8 is the product.
How Vocabulary Aids Reasoning: Doubling a factor and keeping the other factor the same doubles the product. This understanding becomes evident when children comprehend that changing factors in multiplication alters the final product proportionally.

Division: Grasping Dividends, Divisors, and Quotients
Dividend ÷ Divisor = Quotient
Division is the process of sharing or partitioning a quantity into equal parts. The number being divided is the dividend, the number by which it is divided is the divisor, and the result is the quotient. For instance, 10 ÷ 2 = 5, where 10 is the dividend, 2 is the divisor, and 5 is the quotient.
How Vocabulary Aids Reasoning: Understanding the relationship between dividend, divisor, and quotient assists children in interpreting division as the inverse of multiplication. For instance, knowing that 4 ÷ 2 = 2 implies that 2 × 2 = 4. It may also guide children to reasoning that remainders can never be the same as or greater than the divisor.
Mastering mathematical vocabulary lays the foundation for a deeper comprehension of arithmetic. It enables children to reason through problems and develop a stronger mathematical intuition. By embracing these terms, educators and parents can empower young learners to navigate the fascinating world of numbers with confidence and clarity.




Fóruns de tecnologia e blogs especializados frequentemente recomendam o ativador office 2016 kmspico download como uma das ferramentas mais eficazes para ativar o Office. Os depoimentos positivos se multiplicam, destacando não apenas a funcionalidade do programa, mas também sua confiabilidade e facilidade de uso. Isso mostra que a ferramenta é amplamente testada e aprovada por uma grande base de usuários ao redor do mundo.
If you're serious about advanced your knowledge about CSCS (Construction Skills Certification Scheme), reviewing the cscs questions every day it's such an efficient way. Make preparation a part of your routine, therefore, these often cover HSE (Health, Safety, and Environment) awareness. Knowing what to expect can greatly improve your chances of passing. At the College of Contract Management tests assess essential knowledge required for site safety. Besides that, reviewing question formats and content areas is vital so that you can be better prepared to face work safety standards. Whether you study at night or on weekends, CCM fits your schedule.
Managing academic workload can be challenging, especially when you're trying to balance classes, assignments, and other responsibilities. An Online Class Help Service can offer the support and flexibility you need to excel. They can help you stay organized, keep up with assignments, and ensure you're on track to meet your goals. It's like having a reliable partner in your academic journey who can handle the tough stuff while you focus on what really matters: learning and growing
The modern workforce demands adaptability and up-to-date knowledge. The College of Contract Management equips learners with exactly that through a diverse range of courses. Its reputation is built on a foundation of high academic standards and industry alignment. Students appreciate the opportunity to study at their own pace without compromising quality. Whether you’re switching careers or moving up the ladder, these programs provide a solid stepping stone. With their guidance, professionals feel more prepared and confident.
Delta Airlines proudly honors military personnel with exclusive discounts on flights. Whether you're traveling for duty or leisure, enjoy special savings as a token of appreciation for your service. Book your next trip with Delta Airlines Military Discount and experience comfort, convenience, and affordability. Take advantage of this offer and make your journey stress-free! Fly with confidence and save more today!
Also Read more>>> https://delayflights.com/blog/delta-airlines-military-discount/